Article Sidebar

Download
PDF
Statistic
Read Counter : 159 Download : 6

Downloads

Download data is not yet available.

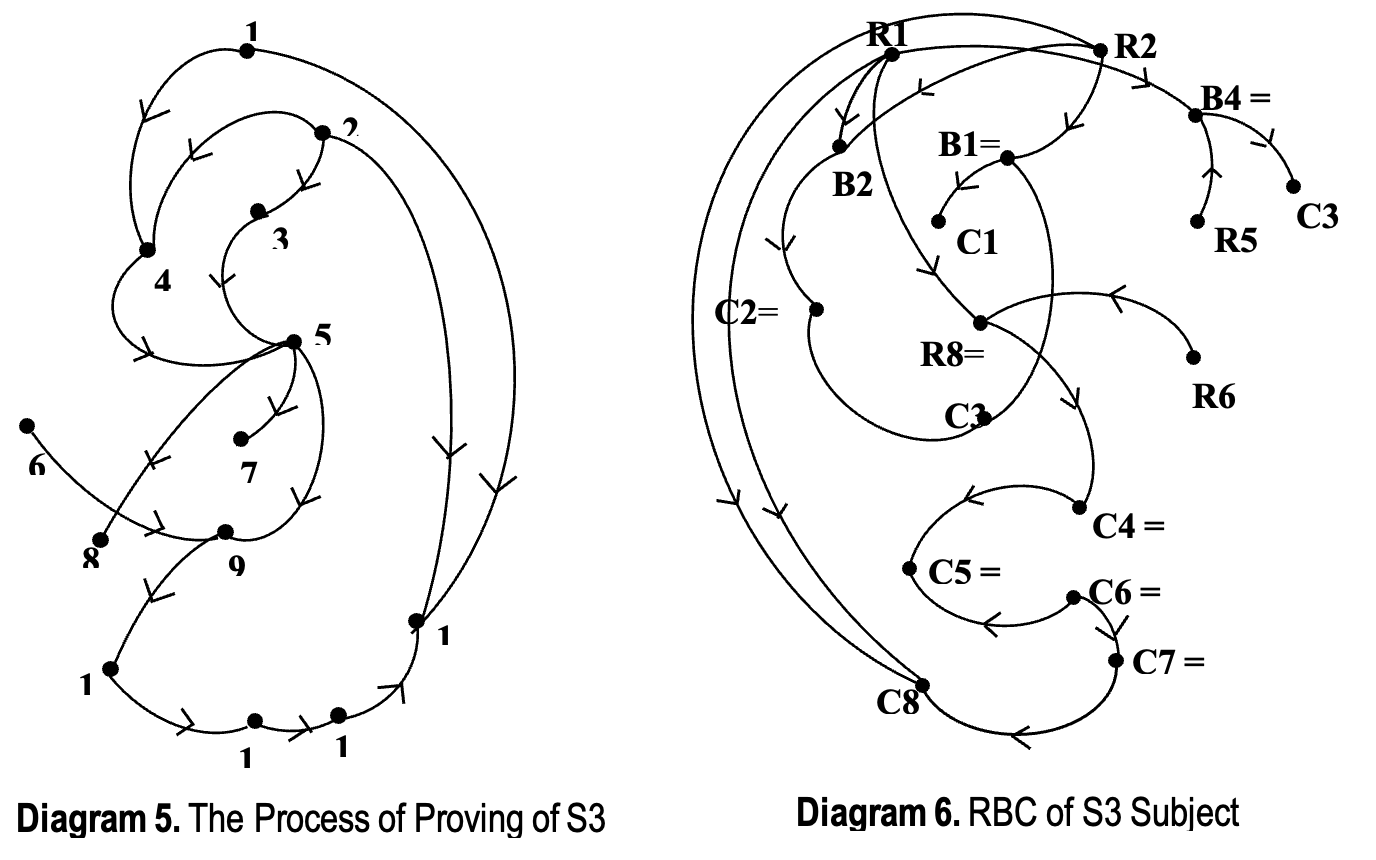
Main Article Content

Abstract

Mathematical abstraction is essential in constructing mathematical concepts, particularly in proof. The RBC+C epistemic actions—recognizing, building-with, constructing, and consolidating—are key cognitive processes in proof construction. However, the impact of mathematical reading and writing abilities on these processes remains unexplored. This study investigates how students’ mathematical reading and writing abilities affect their epistemic actions when proving the congruence of two triangles. This qualitative research adopts a case study design involving three undergraduate students who have completed a geometry course. The participants were selected based on their reading and writing proficiency levels: high, moderate, and low. Data were collected through reading and writing assessments, proof-solving tasks, and semi-structured interviews. The analysis follows the RBC+C framework to identify patterns in students’ cognitive process during proof construction. Findings reveal that students with high mathematical reading and writing abilities demonstrate a more structured proof strategy, effectively recognizing key properties, building logical connections, and constructing valid arguments. High-proficiency students also exhibit flexibility in using both geometric and algebraic approaches in proving. In contrast, students with lower reading and writing abilities struggle with symbolic representation, logical coherence, and notation consistency, leading to incomplete or incorrect proofs. Moreover, consolidation of mathematical ideas, such as reusing known theorems and revisiting proof steps, occurs more frequently in high-achieving students, enabling deeper conceptual understanding. This study highlights the critical role of mathematical literacy in the proof process. It suggests that strengthening reading and writing instruction in mathematics education can enhance students’ ability to construct rigorous proofs. The findings contribute to the development of instructional strategies that integrate mathematical literacy into proof-based learning, ultimately fostering students’ reasoning and problem-solving skills in mathematics.

Keywords

Abstraction Epistemic Action Geometry Mathematical Reading Ability Mathematical Writing Ability

Article Details

Creative Commons License

This work is licensed under a Creative Commons Attribution 4.0 International License.

How to Cite
Setianingsih, R., Budiarto, M. T., & Jamil, A. F. (2025). Epistemic actions in proving two-triangle problems by considering mathematical reading and writing ability. Journal on Mathematics Education, 16(2), 479–496. https://doi.org/10.22342/jme.v16i2.pp479-496
Download Citation
Endnote/Zotero/Mendeley (RIS)
BibTeX

References

  1. Battista, M. T. (1990). Spatial visualization and gender differences in high school geometry. Journal for Research in Mathematics Education, 21(1), 47–60. https://doi.org/10.2307/749456
  2. Baxter, J. A., Woodward, J., & Olson, D. (2005). Writing in mathematics: An alternative form of communication for academically low-achieving students. Learning Disabilities Research & Practice, 20(2), 119–135. https://doi.org/10.1111/j.1540-5826.2005.00127.x
  3. Bicer, A., Capraro, R. M., & Capraro, M. M. (2013). Integrating writing into mathematics classroom to increase students’ problem solving skills. International Online Journal of Educational Sciences, 5(2), 361–369. https://www.researchgate.net/publication/281466142
  4. Breive, S. (2022). Abstraction and embodiment: Exploring the process of grasping a general. Educational Studies in Mathematics, 110(2), 313–329. https://doi.org/10.1007/s10649-021-10137-x
  5. Çakiroğlu, Ü., & Çevik, İ. (2022). A framework for measuring abstraction as a sub-skill of computational thinking in block-based programming environments. Education and Information Technologies, 27(7), 9455–9484. https://doi.org/10.1007/s10639-022-11019-2
  6. Cooper, T. E. (2012). Using virtual manipulatives with pre-service mathematics teachers to create representational models. International Journal for Technology in Mathematics Education, 19(3), 105-116. http://www.researchinformation.co.uk/timearch/2012-03/pageflip.html
  7. Dreyfus, T., Hershkowitz, R., & Schwarz, B. (2002). Abstraction in context II: The case of peer interaction. Cognitive Science Quarterly, 1(3/4), 307–368. https://www.researchgate.net/publication/273134154
  8. Dreyfus, T., Hershkowitz, R., & Schwarz, B. (2015). The nested epistemic actions model for abstraction in context: Theory as methodological tool and methodological tool as theory. In A. Bikner-ahsbahs, C. Knipping, & N. Presmeg (Eds.), Approaches to Qualitative Research in Mathematics Education (pp. 185–217). Springer. https://doi.org/10.1007/978-94-017-9181-6_8
  9. Dreyfus, T., & Kidron, I. (2014). Introduction to abstraction in context (AiC). Networking of Theories as a Research Practice in Mathematics Education, 85–96. https://doi.org/10.1007/978-3-319-05389-9_6
  10. Dreyfus, T., & Tsamir, P. (2004). Ben’s consolidation of knowledge structures about infinite sets. The Journal of Mathematical Behavior, 23(3), 271–300. https://doi.org/10.1016/j.jmathb.2004.06.002
  11. Ferrari, P. L. (2003). Abstraction in mathematics. Philosophical Transactions of the Royal Society B: Biological Sciences, 358(1435), 1225–1230. https://doi.org/10.1098/rstb.2003.1316
  12. Fitriani, N., Suryadi, D., & Darhim, D. (2018). The students’mathematical abstraction ability through realistic mathematics education with vba-microsoft excel. Infinity Journal, 7(2), 123–132. https://e-journal.stkipsiliwangi.ac.id/index.php/infinity/article/view/833
  13. Freitag, M. (1997). Reading and writing in the mathematics classroom. The Mathematics Educator, 8(1). https://openjournals.libs.uga.edu/tme/article/view/1704
  14. Gilboa, N., Kidron, I., & Dreyfus, T. (2019). Constructing a mathematical definition: The case of the tangent. International Journal of Mathematical Education in Science and Technology, 50(3), 421–446. https://doi.org/10.1080/0020739X.2018.1516824
  15. Grossman, F. J., Smith, B., & Miller, C. (1993). Did you say" write" in mathematics class? Journal of Developmental Education, 17(1), 2. https://eric.ed.gov/?id=EJ469265
  16. Gullatt, D. E. (1986). Help your students read mathematics. The Arithmetic Teacher, 33(9), 20–21. https://doi.org/10.5951/AT.33.9.0020
  17. Harris, M. J., & VanDevender, E. M. (1990). Overcoming the confusion of reading mathematics. Focus on Learning Problems in Mathematics, 12(1), 19–27. https://eric.ed.gov/?id=EJ419484
  18. Hershkowitz, R., Dreyfus, T., & Schwarz, B. B. (2020). Abstraction in context. Encyclopedia of Mathematics Education, 9–13. https://doi.org/10.1007/978-3-030-15789-0_100032
  19. Hershkowitz, R., Schwarz, B. B., & Dreyfus, T. (2001). Abstraction in context: Epistemic actions. Journal for Research in Mathematics Education, 32(2), 195–222. https://doi.org/10.2307/749673
  20. Hong, J. Y., & Kim, M. K. (2016). Mathematical abstraction in the solving of ill-structured problems by elementary school students in Korea. Eurasia Journal of Mathematics, Science and Technology Education, 12(2), 267–281. https://doi.org/10.12973/eurasia.2016.1204a
  21. Jirotková, D., & Littler, G. H. (2005). Classification leading to structure. Building Structures in Mathematical Knowledge, 5, 321–331. https://www.academia.edu/390934/Building_structures_in_mathematical_knowledge
  22. Jones, K. (2002). Issues in the teaching and learning of geometry. In Aspects of teaching secondary mathematics (pp. 137–155). Routledge. https://doi.org/10.4324/9780203165874-14
  23. Jones, K., Fujita, T., & Miyazaki, M. (2013). Learning congruency-based proofs in geometry via a web-based learning system. Proceedings of the British Society for Research into Learning Mathematics, 33(1), 31–36. https://eprints.soton.ac.uk/372524/1/Jones-etc_learning_congruency_proofs_web-based_2013.pdf
  24. Kesorn, N., Junpeng, P., Marwiang, M., Pongboriboon, K., Tang, K. N., Bathia, S., & Wilson, M. (2020). Development of an assessment tool for mathematical reading, analytical thinking and mathematical writing. International Journal of Evaluation and Research in Education, 9(4), 955–962. https://eric.ed.gov/?id=EJ1274748
  25. Kim, H. J., Choi, W.-H., & Lee, Y. (2020). Construction of reversible self-dual codes. Finite Fields and Their Applications, 67, 101714. https://doi.org/10.1016/j.ffa.2020.101714
  26. King, B., Raposo, D., & Gimenez, M. (2016). Promoting student buy-in: Using writing to develop mathematical understanding. Georgia Educational Researcher, 13(2), 33. https://doi.org/10.20429/ger.2016.130202
  27. Levenberg, I., & Shaham, C. (2014). Formulation of word problems in geometry by gifted pupils. Journal for the Education of Gifted Young Scientists, 2(2), 28–40. https://dergipark.org.tr/en/pub/jegys/issue/37434/432907
  28. Magiera, M. T., & Zawojewski, J. S. (2011). Characterizations of social-based and self-based contexts associated with students’ awareness, evaluation, and regulation of their thinking during small-group mathematical modeling. Journal for Research in Mathematics Education, 42(5), 486–520. https://doi.org/10.5951/jresematheduc.42.5.0486
  29. Österholm, M. (2006). A reading comprehension perspective on problem solving. MADIF 5, the 5th Swedish Mathematics Education Research Seminar, January 24-25, Malmö, Sweden, 136–145. https://www.researchgate.net/publication/253463462_A_Reading_Comprehension_Perspective_on_Problem_Solving
  30. Park, M., & Lee, K.-H. (2022). A case study on the relationship between indefinite integral and definite integral according to the AiC perspective. Communications of Mathematical Education, 36(1), 39–57. https://doi.org/10.7468/jksmee.2022.36.1.39
  31. Retnawati, H., Djidu, H., Kartianom, Apino, E., & Anazifa, R. D. (2018). Teachers’ knowledge about higher-order thinking skills and its learning strategy. Problems of Education in the 21st Century, 76(2), 215–230. https://doi.org/10.33225/pec/18.76.215
  32. Sahrudin, A., Budiarto, M. T., & Manuharawati, M. (2022). Epistemic action of junior high school students with low spatial ability in constructing cube nets. International Journal of Educational Methodology, 8(2), 221–230. https://doi.org/10.12973/ijem.8.2.221
  33. Shibli, A. (1992). Increasing learning with writing in quantitative and computer courses. College Teaching, 40(4), 123–127. https://www.jstor.org/stable/27558551
  34. Sipka, T. (1990). Writing in mathematics: A plethora of possibilities. In A. Sterrett (Ed.), Using writing to teach mathematics (pp. 11–14). Mathematical Association of America Washington, DC. https://www.readingrockets.org/topics/writing/articles/integrating-writing-and-mathematics
  35. Smith, B., Miller, C. A., & Grossman, F. J. (1992). Comprehending mathematical concepts: Relating reading and writing to mathematical performance. Journal of College Reading and Learning, 25(1), 51–64. https://eric.ed.gov/?id=EJ457223
  36. Sunzuma, G., & Maharaj, A. (2019). In-service teachers’ geometry content knowledge: Implications for how geometry is taught in teacher training institutions. International Electronic Journal of Mathematics Education, 14(3), 633–646. https://doi.org/10.29333/iejme/5776
  37. Tall, D. (1991). Advanced mathematical thinking (Vol. 11). Springer Science & Business Media. https://doi.org/10.1007/0-306-47203-1
  38. Teuscher, D., Kulinna, P. H., & Crooker, C. (2015). Writing to learn mathematics: An update. The Mathematics Educator, 24(2), 56–78. https://doi.org/10.63301/tme.v24i2.2004
  39. Wallace, F. H., & Clark, K. K. (2005). Reading stances in mathematics: Positioning students and texts. Action in Teacher Education, 27(2), 68–79. https://doi.org/10.1007/978-94-007-1174-7
  40. Woolley, G., & Woolley, G. (2011). Reading comprehension. Springer.
  41. Yang, K.-L. (2016). Analyzing mathematics textbooks through a constructive-empirical perspective on abstraction: The case of Pythagoras’ theorem. Eurasia Journal of Mathematics, Science and Technology Education, 12(4), 913–930. https://doi.org/10.12973/eurasia.2016.1237a